Antenna Position

Obtaining u,v,w From An Antenna Array

A synthesis imaging radio instrument consists of a number of radio elements (radio dishes, dipoles, or other collectors of radio emission), which represent measurement points in u,v,w space. We need to describe how to convert an array of dishes on the ground to a set of points in u,v,w space.

E, N, U coordinates to x, y, z

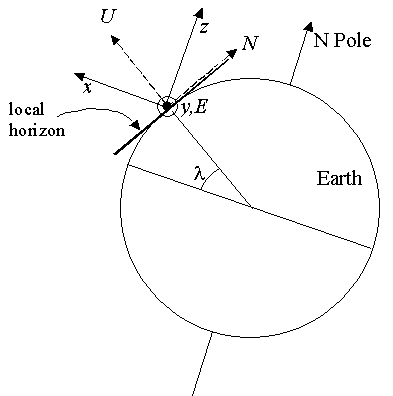
The first step is to determine a consistent coordinate system. Antennas are typically measured in units such as meters along the ground. We will use a right-handed coordinate system of East, North, and Up (E, N, U). These coordinates are relative to the local horizon, however, and will change depending on where we are on the spherical Earth. It is convenient in astronomy to use a coordinate system aligned with the Earth's rotational axis, for which we will use coordinates (x, y, z) as shown in Figure 1. Conversion from (E, N, U) to (x, y, z) is done via a simple rotation matrix:

Fig. 1: The relationship between E, N, U coordinates and x, y, z coordinates, for a latitude Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} . The direction of z is parallel to the direction to the celestial pole. The directions y and E are the same direction.

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{bmatrix} x \\ y \\ z \\ \end{bmatrix} = \begin{bmatrix} 0 & -\sin \lambda & \cos \lambda \\ 1 & 0 & 0\\ 0 & \cos \lambda & \sin \lambda \\ \end{bmatrix} \begin{bmatrix} E \\ N \\ U \\ \end{bmatrix} }

which yields the relations:

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} x & = -N \sin \lambda + U \cos \lambda \\ y & = E \\ z & = N \cos \lambda + U \sin \lambda \end{align} }

Baselines and Spatial Frequencies

Note that the baselines are differences of coordinates, i.e. for the baseline between two antennas we have a vector:

Fig. 2: Geometry of an interferometer baseline where a delay Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tau} is inserted in one antenna, in order to steer the phase center to a direction $\theta _{o}$ from the vertical Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} .

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{B} = (B_x, B_y, B_z) = (x_2 - x_1, y_2 - y_1, z_2- z_1)}

This vector difference in positions can point in any direction in space, but the part of the baseline that matters in calculating u,v,w is the component perpendicular to the direction Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta _o} (the phase center direction), which we called Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B_{proj}} in Figure 2. Let us express the phase center direction as a unit vector Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{s_o}} Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle = (h_o, \delta_o)} , where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h_o} is the hour angle (relative to the local meridian) and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta_o} is the declination (relative to the celestial equator). Then Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B_{proj} = \vec{B} \cdot \vec{s_o}= B \cos \theta _o} .

Recall that the spatial frequencies u,v,w are just the distances expressed in wavelength units, so we can get the u,v,w coordinates from the baseline length expressed in wavelength units from the following coordinate transformation (see Thompson 1999 for details):

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{bmatrix} u \\ v \\ w \\ \end{bmatrix} = \vec{B_{\lambda}} \cdot \vec{s_o} = (1/\lambda) \begin{bmatrix} \sin h_o & \cos h_o & 0 \\ - \sin \delta _o \cos h_o & \sin \delta _o \sin h_o & \cos \delta _o\\ \cos \delta _o \cos h_o & - \cos \delta _o \sin h_o & \sin \delta _o \\ \end{bmatrix} \begin{bmatrix} B_x \\ B_y \\ B_z \end{bmatrix} } Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (1)}

How baseline errors can contribute to the error in phase

The geometric phase difference at the phase center (Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle w} term in (1)) is:

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi_g = 2\pi\tau_g\nu = (2\pi/\lambda)[B_x\cos \delta_o \cos h_o - B_y\cos \delta_o\sin h_o + B_z\sin \delta_o]}

where Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tau _g = \vec{B} \cdot \vec{s} /c} , geometric delay. We can see what can affect the geometric phase by taking the differential of this expression:

Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} d\phi_g = 2\pi\nu d\tau_g = (2\pi/\lambda)[&dB_x\cos \delta_o \cos h_o - dB_y\cos \delta_o \sin h_o + dB_z\sin \delta_o \\ +\ &d\alpha_o\cos \delta_o (B_x\sin h_o + B_y\cos h_o) \\ +\ &d\delta_o (-B_x\cos h_o \sin \delta_o + B_y\sin h_o \sin \delta_o + B_z\cos \delta_o)]\end{align}} $(2)$

where we have used the relation between right ascension and hour angle: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h_o = LST - \alpha_o} , so Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dh_o = -d\alpha_o} . Equation (2) shows how baseline errors Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (dB_x, dB_y, dB_z)} and source position errors (Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha_o} , Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta_o} ) will affect the error in group delay Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\tau_g} (or yield an error in phase Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\phi_g} ). Note that a clock error is equivalent to a source position error Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\alpha_o} .

If we have a source whose position is known, we can use Equation (2) to find the location of the antennas (this is called baseline determination). The error in antenna position is largely independent of the baseline lengths. For example, say that we can measure Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\phi_g} to within 1 degree at 5 GHz (Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} = 6 cm). Then we can measure Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dB_x} , Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dB_y} and Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dB_z} to a precision of order (1 / 360) 6 cm ~ 1 / 60 cm even though Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B = (B_x^2 + B_y^2 + B_z^2)^{1/2}} = 5000 km or more (VLBI).

The time of day and location of the antennas must be known to relatively high accuracy -- needed for determining the geometric delay. A clock error of 1 s, or a baseline error of a few cm, will cause a serious phase shift of the source over, say, 10 minutes. At OVRO, using a GPS clock and measuring baselines with cosmic source calibration, we get a time accuracy of << 1 ms, and baseline errors of about 3 mm. Therefore, these effects are not serious over a short time interval, but may still be problematic over 8 hours. This is one reason that we do phase calibration observations every ~ 2 hours.